

Previous: Experiment 6: Propagation and optical signal processing
Next: Experiment 8: Polarization
Subsections
Light waves incident on the sharp edge of an opaque object exhibit an
effect called diffraction. This phenomenon cannot be explained by the
geometric theory of light that predicts a simple shadow.
Expand the laser beam with a Galilean telescope (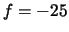 mm and
mm and
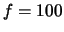 mm). Position the aperture mask such that half of the beam is
blocked by one edge of the aperture. Observe the behavior of the beam
after the edge. Note in particular the distribution right after the
edge and far from it.
mm). Position the aperture mask such that half of the beam is
blocked by one edge of the aperture. Observe the behavior of the beam
after the edge. Note in particular the distribution right after the
edge and far from it.
Expand the laser beam with a Galilean telescope (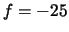 mm and
mm and
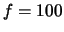 mm). Position the slide containing single slits of various
widths in the beam path. Use the aperture mask to select an individual
slit. Observe the changes in the diffraction pattern as the light
propagates away from the slit. In the far field, measure the distance
between the zeros on either side of the main lobe. Calculate the slit
width from this measurement and compare with the given value. What do
you think is the functional dependence of the intensity on the horizontal
axis? Why do you see diffraction only in the horizontal plane? How
far do you have to be from the slit for the far field approximation to
be valid? Repeat for all the slits on the slide.
mm). Position the slide containing single slits of various
widths in the beam path. Use the aperture mask to select an individual
slit. Observe the changes in the diffraction pattern as the light
propagates away from the slit. In the far field, measure the distance
between the zeros on either side of the main lobe. Calculate the slit
width from this measurement and compare with the given value. What do
you think is the functional dependence of the intensity on the horizontal
axis? Why do you see diffraction only in the horizontal plane? How
far do you have to be from the slit for the far field approximation to
be valid? Repeat for all the slits on the slide.
Position the 80 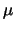 m circular aperture on the laser beam. (Do not
expand the beam.) Observe the changes in the diffraction pattern as the
light propagates away from the aperture. In the far field, measure the
distance between the zeros on either side of the main lobe. Calculate
the aperture diameter from this measurement and compare with the given
value. Repeat for the 40
m circular aperture on the laser beam. (Do not
expand the beam.) Observe the changes in the diffraction pattern as the
light propagates away from the aperture. In the far field, measure the
distance between the zeros on either side of the main lobe. Calculate
the aperture diameter from this measurement and compare with the given
value. Repeat for the 40 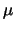 m circular aperture.
m circular aperture.
The theory of diffraction gratings is outlined on pages 60 through 62
of the textbook. Verify Equation (2.4-10) for an incidence angle of
zero using the laser beam. The diffraction grating has 528 lines/mm.
Position the diffraction grating right after a single slit illuminated
by the laser. Observe the resulting diffraction pattern. Which Fourier
transform theorem does this experiment illustrate?
Create a diverging Gaussian beam by positioning a negative lens
(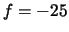 mm) as close to the laser as possible. Position the
transmission hologram in the beam path at an incidence angle of
approximately 60
mm) as close to the laser as possible. Position the
transmission hologram in the beam path at an incidence angle of
approximately 60 . Make sure that the entire hologram is
illuminated. View the hologram from the side.
. Make sure that the entire hologram is
illuminated. View the hologram from the side.


Previous: Experiment 6: Propagation and optical signal processing
Next: Experiment 8: Polarization
Orhan Aytür
![]() mm and
mm and
![]() mm). Position the aperture mask such that half of the beam is
blocked by one edge of the aperture. Observe the behavior of the beam
after the edge. Note in particular the distribution right after the
edge and far from it.
mm). Position the aperture mask such that half of the beam is
blocked by one edge of the aperture. Observe the behavior of the beam
after the edge. Note in particular the distribution right after the
edge and far from it.
![]()
![]()
![]()