Subsections

• 1 Gaussian intensity profile
• 2 Measurement of beam parameters

Experiment 5: Gaussian beams

The spatial characteristics of a laser beam are determined by the laser resonator (see Chapters 9 and 14). Most lasers have spherical mirror Fabry-Perot resonators that have Hermite-Gaussian spatial modes. Usually, only the lowest order transverse resonator mode oscillates, resulting in a Gaussian output beam. The location and the radius of the beam waist depend on the laser resonator. For the He-Ne lasers used in the Optics Laboratory, you can assume that the beam waist is located at the output aperture of the laser. The diameter of the beam waist is approximately 0.48 mm.

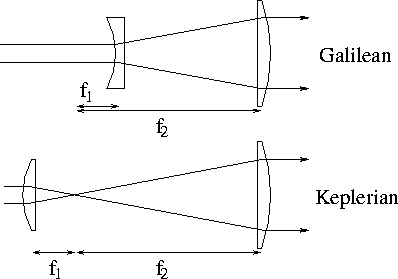
Some applications require a laser beam with low divergence (small 2$\theta_0$). This can be achieved by expanding a laser beam with a telescope so that $W_0$ and $z_0$ become large, resulting in a collimated beam. A Galilean or a Keplerian telescope may be used for this purpose. In both telescopes, the first lens ($f_1$) expands the beam and the second lens ($f_2$) recollimates it.

Some applications require a beam that is focused to a small spot. A single positive lens may be adequate for this purpose. The spot size decreases with increasing beam radius at the focusing lens.

1 Gaussian intensity profile

In this experiment, you will measure the intensity profile of the He-Ne laser beam and verify that it is a Gaussian. This can be done by sampling the intensity along a transverse direction with the help of a circular aperture. This technique is adequate only if the aperture diameter is much smaller than the beam radius at the plane of the measurement.

First expand the laser beam with a Galilean telescope ($f=-25$ mm and $f=400$ mm). Make sure that the resulting beam is well collimated by visually checking that the beam propagates virtually without divergence after the second lens.

Mount the 0.5 mm aperture (one of the light source apertures) on a slide holder. Mount the slide holder onto the translation stage such that the aperture can be positioned anywhere on the transverse plane. Position the translation stage on the optical bench so that the aperture is in the beam path, and visually center the aperture on the beam. Position the optical power meter after the aperture. Screw the He-Ne (red) filter in front of the power meter detector. Make sure that all the light going through the aperture is incident on the detector at each measurement point.

Measure the intensity at 1 mm intervals along the horizontal transverse direction. Plot your data and try to fit a Gaussian curve to it. Determine the beam radius $W$ from your graph. Assuming that the beam waist is located right after the recollimating lens, calculate the beam radius at 100 m, 1 km, and 10 km. Calculate the divergence angle ($2\theta_0$) of this beam. Using the known magnification of the telescope ($\times 16$), calculate the approximate beam radius and divergence before the telescope.

2 Measurement of beam parameters

The phase radius $R$ and beam radius $W$ at any point along the propagation axis $z$ characterize a Gaussian beam completely. However, determining the phase radius experimentally requires a difficult interferometric measurement. Equivalently, we can characterize the beam by measuring $W$ at two different locations along the propagation axis. These measurements allow us to calculate all the beam parameters.

In this experiment you will measure the beam radius for a diverging beam at two positions along the direction of propagation and deduce all the beam parameters from these measurements. Before coming to the laboratory, derive all necessary expressions to deduce $z_0$, $W_0$, $z_1$, $z_2$, $R(z_1)$, and $R(z_2)$ from a knowledge of $W(z_1)$, $W(z_2)$, and $d=z_2-z_1$. Note that you do not know the values of $z_1$ and $z_2$, but only their difference $d$.

In the laboratory, focus the laser beam with a $f=35$ mm lens. Using the 0.5 mm aperture and the translation stage, measure the beam radius at two different $z$ positions $z_1$ and $z_2$ after the focus. (To measure the beam radus, just find the location where the intensity drops down to $1/e^2$ of its peak value rather than measuring the entire beam profile.) Choose $z_1$ and $z_2$ so that the beam is considerably larger than the aperture (0.5 mm) you are using. From your data, find $z_0$, $W_0$, $2\theta_0$, $z_1$, $R(z_1)$, and $R(z_2)$.